This is the last post I'll make in 2013, so it's time to take a look back at the year in posts.

There was a lot to go over this year, so I can't hit every post, but I think you'll enjoy the highlights I've chosen!

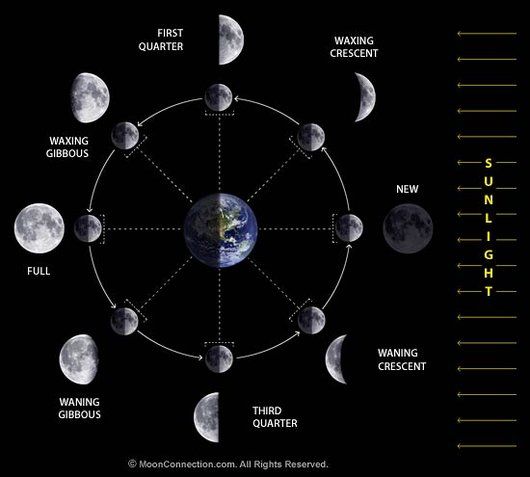
JANUARY: I kicked the year off with the announcement of my new Moon Phase For Any Date tutorial. Closely related to the moon tutorial was the lesson on how to work out the date of Easter for any date.

I also made several happy discoveries, including the release of James Burke's unedited documentaries on YouTube, and a maze programming tutorial I hadn't seen since I was a teenager!

FEBRUARY: February seemed to be a month for mental math tutorials. Besides a few quick feats, you learned about verifying prime numbers mentally, and the unusual technique of leapfrog division. Just for fun, we even brought Pi digits to life!

MARCH: We celebrated Grey Matters' 8th blogiversary with the most posts of any month in 2013! There were several offbeat memory challenges, and numerous offbeat math lessons, such as continued fractions, getting the most out of a single equation, untangling ropes mathematically, and even Scam School's lessons about cube roots.

APRIL: This month was quiet, but we still managed to cover new uses for old calculators. With Scam School's help, there were even lessons on memorizing a list of 20 items and an impressive mobile phone magic routine!

MAY: We returned to the basics at this point in the year. YouTublerone's memory technique videos made taught in a vivid way, and mental adjustments between the Gregorian and Julian calendars were made clearer. Learning to demonstrate mental feats in an entertaining way was also on the agenda.

JUNE: James Grime shared a fun way to predict sums, and Mel Stover's work was finally given due respect on Grey Matters. An interesting lesson on how to remember and calculate temperature conversions was also one of June's highlights.

JULY: After the previous month's temperature conversion post, a few new additions were introduced to make this easier. Multiplying by ¹⁰⁄₉ was made simpler, and a technique developed for this was expanded to make numerous other mental math feats easier. Our favorite game Nim also returned.

AUGUST: The main focus of this month was a mystery feat! First, a technique for mentally multiplying by 63 was taught, followed immediately by how to mentally multiply by 72. What skill could possibly require these two abilities? I won't ruin it for you, but the answer can be found here.

SEPTEMBER: Just like May dealt with the basics, September brought complex concepts down to Earth, literally, in some cases. Quick magic tricks were an early treat, and Mental Floss' Be More Interesting gallery presented an entire buffet of techniques. Logarithms and astronomy were also explored and clarified.

OCTOBER: Picking up from September, memorizing the elements, and understanding them better with help from TED-Ed videos was the order of the day. Untangling family trees with help from Wolfram Alpha was another challenge we took on. Once again, Nim, this time in finger-dart form, put in another appearance on Grey Matters.

NOVEMBER: As the holiday season drew near, Dr. Arthur Benjamin seemed to take over the blog. His performance and lecture about mental math techniques was released shortly before his fun lecture about fibonacci numbers. Inspired by this, I also taught how to work out 3-digit cube roots, along with some handy tips and tricks.

DECEMBER: As the year drew to a close, I paused for a post about time, space, and perspective, and looked back at some classic programs that helped clarify history, math, and more. The Pebbling The Chessboard problem rounded out the year with a good look at how to turn a problem into its mathematical equivalent.

Now, it's time to start looking forward to 2014, when we'll wind up 9 years of posts, and begin working on the 10th! I hope you'll join me here on Grey Matters!

It's not always easy to see how the mathematics of a problem connects to the problem itself.

In this post, with a little help from NumberPhile and Mills College mathematics professor Zvezdelina Stankova, you're going to learn about an unusual chessboard problem, and how the physical problem is turned into mathematics!

The problem starts with a chessboard that stretches off into infinity. A “prison” is marked off and some unusual rules are set, as explained in the following video. You might want to stop the video around the 3-minute mark, and try the challenge for yourself.



Now that you've got the basic idea of this puzzle, can you work out what would happen if you used different starting arrangements of clones? What about in “prisons” of different sizes? Professor Stankova continues with several variations in another related video:



After discussing how to handle variations, Professor Stankova then goes on to discuss a more general version of the problem, from which prisons can the clones escape, and from which ones can they NOT escape? You can read the original paper referred to in this third video online for free (PDF), if this problem is starting to grab your interest.

There's even a fourth video, largely consisting of footage excerpted from the first video above, in which Professor Stankova talks about how a problem is proved impossible when that happens to be the case.

Even if you don't find the particular problem intriguing, I still believe these videos are worth viewing in detail, as you get a good concept of overall problem solving and representing a problem mathematically in particular.

Note: This post first appeared on Grey Matters in 2007. Since then, I've made it a sort of annual tradition to post it every December, with the occasional update. Enjoy!

Since the focus of this blog is largely math and memory feats, it probably won't be a surprise to learn that my favorite Christmas carol is The 12 Days of Christmas. After all, it's got a long list and it's full of numbers!

On the extremely unlikely chance you haven't heard this song too many times already this holiday season, here's John Denver and the Muppets singing The 12 Days of Christmas:



The memory part is usually what creates the most trouble. In the above video, Fozzie has trouble remembering what is given on the 7th day. Even a singing group as mathematically precise as the Klein Four Group has trouble remembering what goes where in their version of The 12 Days of Christmas (Their cover of the Straight No Chaser version):



Just to make sure that you've got them down, I'll give you 5 minutes to correctly name all of the 12 Days of Christmas gifts. Those of you who have been practicing this quiz since I first mentioned it back in 2007 will have an advantage.

Now that we've got the memory part down, I'll turn to the math. What is the total number of gifts are being given in the song? 1+2+3 and so on up to 12 doesn't seem easy to do mentally, but it is if you see the pattern. Note that 1+12=13. So what? So does 2+11, 3+10 and all the numbers up to 6+7. In other words, we have 6 pairs of 13, and 6 times 13 is easy. That gives us 78 gifts total.

As noted in Peter Chou's Twelve Days Christmas Tree page, the gifts can be arranged in a triangular fashion, since each day includes one more gift than the previous day. Besides being aesthetically pleasing, it turns out that a particular type of triangle, Pascal's Triangle, is a great way to study mathematical questions about the 12 days of Christmas.

First, let's get a Pascal's Triangle with 14 rows (opens in new window), so we can look at what it tells us. As we discuss these patterns, I'm going to refer to going down the right diagonal, but since the pattern is symmetrical, the left would work just as well.

Starting with the rightmost diagonal, we see it is all 1's. This represents each day's increase in the number of presents, since each day increases by 1. Moving to the second diagonal from the right, we see the simple sequence 1, 2, 3, 4, 5, 6, 7, 8, 9, 10, 11, and 12, which can naturally represent the number of gifts given on each day of Christmas.

The third diagonal from the right has the rather unusual sequence of 1, 3, 6, 10, 15, 21, 28, 36, 45, 55, 66, 78, 91. This is a pattern of triangular numbers.

But what can triangular numbers tell us about the 12 days of Christmas? If you look at where the 3 in this diagonal, it's southwest (down and to the left) of the 2 in the second rightmost diagonal. If, on the 2nd day of Christmas, you gave 2 turtle doves and 1 partridge in a pear tree, you would indeed have given 3 gifts, but does the pattern hold? On the 3rd day, you would have given 3+2+1 (3 French hens, 2 turtle doves and a partridge in a pear tree) or 6 gifts total, and sure enough, 6 can be found southwest of the 3! For any of the 12 days, simply find that number, and look to the southwest of that number to see how many gifts you've given by that point! Remember when figured out that the numbers 1 through 12, when added, totaled 78? Look southwest of the 12, and you'll find that same 78!

Let's get really picky and technical about the 12 days of Christmas. It clearly states that on the first day, your true love gave you a partridge in a pear tree, and on the second day your true love gave you two turtle doves and a partridge in a pear tree. You would actually have 4 gifts (counting each partridge and its respective pear tree as one gift) by the second day, the first day's partridge, the second day's partridge and two turtle doves. By the third day, you would have 10 gifts, consisting of 3 partridges, 4 turtle doves and 3 French hens.

At this rate, how many gifts would you have at the end of the 12th day? Sure enough, the pattern of 1, 4, 10 and so on, known as tetrahedral numbers, can be found in our Pascal's Triangle as the 4th diagonal from the right.

If you look at the 2nd rightmost diagonal, you'll see the number 2, and you'll see the number 4 two steps southwest (two steps down and to the left) of it, which tells us you'll have 4 gifts on the second day. Using this same method, you can easily see that you'll have 10 gifts on the 3rd day, 20 gifts on the 4th day, and so on. If you really did get gifts from your true love in this picky and technical way, you would wind up with 364 gifts on the 12th day! In other words, you would get 1 gift for every day in the year, not including Christmas itself (also not including February 29th, if we're talking about leap years)!

If you're having any trouble visualizing any of this so far, Judy Brown's Twelve Days of Christmas and Pascal's Triangle page will be of great help.

One other interesting pattern I'd like to bring up is the one that happens if you darken only the odd-numbered cells in Pascal's Triangle. You get a fractal pattern known as the Sierpinski Sieve. No, this won't tell you too much about the 12 days of Christmas, except maybe the occurrences of the odd days, but it can make a beautiful and original Christmas ornament! If you have kids who ask about it, you can always give them the book The Number Devil, which describes both Pascal's Triangle and Sierpinski Sieve, among other mathematical concepts, in a very kid-friendly way.

There's another 12 Days of Christmas calculation that's far more traditional: How much would the 12 gifts actually cost if you bought them? PNC has been doing their famous Christmas Price Index since 1986, and has announced their results. Rather than repeat it here, check out their site and help them find all 12 gifts, so that you can some holiday fun and then find out the total!

Since my Christmas spending is winding up, I'm going to have to forgo the expensive version, in favor of Miss Cellania's internet-style version of The 12 Days of Christmas. Happy Holidays!

It's time for December's snippets.

I've noticed the simpler, more direct skills prove popular, so this month will feature more skills you can learn, use, and demonstrate quickly.

• We'll start off with a simple skill: figuring out your longitude by looking at the night sky, assuming you're in the northern hemisphere. First, you need to find the star Polaris, which is why you need to be in the northern hemisphere for this to work. If you don't know how to do that, my post from September about learning to find various stars will be of help here.

The next step is to determine how many degrees above the horizon Polaris is located. This post from One Minute Astronomer shows how to measure the approximate angle using only your hands! This is a fun skill to demonstrate and teach, as well.

• From arrangements of stars, we come down to earth to arrangements of numbers. Michael Daniels, over at mindmagician.org, has posted a new magic square generator which can handle any integer from 34 through 9999. If you're curious about the method used to create these, you can learn more about it in his ebook, Mostly Perfect. You can even download free excerpts from the book for free!

• One of my favorite feats, the calendar feat, is taught in a very simple and direct version in the following video from Mister Numbers:



If you're not already familiar with Mister Numbers' work on YouTube, check out his channel, and see some of his other work in number patterns. He details more about this calendar procedure in his Kindle ebook, Amazing Calendar Math Magic.

This method has it roots in John Conway's Doomsday Method, and I show how to build on this basis in a simple way to handle almost any year in my ebook, Day One.

• Also from Mister Numbers, here's an impressive video that quickly teaches kids, or anyone really, to be able to handle multiplying the numbers from 1 to 40, and beyond, by themselves in a simple way:



I take advantage of this same basic pattern in my lessons on extracting the roots of perfect squares over in the Mental Gym, so this is a very useful pattern to know!

I hope you've found something quick an interesting. Have any quick and interesting math tips or patterns of your own? I'd love to hear about them in the comments!

Growing up, I'd run into educational videos that caught my interest with creative approaches that really helped me remember the concepts.

In this post, I'd like to share several of these videos which have been made available online for free.

JAMES BURKE VIDEOS: I posted about this at the beginning of the year, but I can't recommend James Burke's documentaries too many times.

Back in the late '70s and early '80s, when history was still being taught in the traditional linear manner, James Burke's approach of teaching history in a more real-world, zig-zag style that made it entertaining, and easier to grasp and understand. This zig-zag approach may seem standard to a generation growing up on clicking links on the internet, but in a world where a wide web was still 10-20 years into he future, this was groundbreaking.

About a year ago, the James Burke Web Channel posted the uncut versions of James Burke's video for the first time. If you're new to James Burke's work, I suggest starting where the rest of the world did, with the original series, Connections.



EUREKA!: Physics can often seem to be the most rigorous and boring subject. The series Eureka! (not to be confused with the US or UK series with similar names) managed to add a bit of fun by turning the lessons into 5-minute animated shorts. The narrator would describe the basics, occasionally interacting with an onscreen animated character who only communication via sounds that demonstrated surprise or understanding. It's a fun little series, as you can see by the first lesson below:



TOTAL BREEZE MATHEMATICS: I just mentioned this a few posts ago, but it also bears repeating here. This is an instructional series on mental math techniques that was originally posted on the web by its creators, but then disappeared quickly. Most of the original videos have been reposted online.



The videos below are only available in Flash format, which is usually bad news for people browsing the web from mobile devices which don't use the Flash plug-in. However, through personal experimentation, I have found the Puffin Browser (available for iOS and Android) capable of displaying these videos with little problem.

PROJECT MATHEMATICS!: I first posted about this series in mid-2012, but the videos were scattered across the web, and weren't all available. Since I've posted, many of those videos have been removed from the web.

For the first time, I've found the complete set of Project Mathematics! videos all in one place on the web! Back in the 1980s, California Technical Institute (CalTech) used computer animation and multimedia in a way that had never been seen before, in order to simplify the teaching of high school mathematics concepts. Fun animations of things like a hand cranks to change the values of numbers to show their effects on the math made these subjects much easier to grasp.

The full list of episodes is here, along with an overview of each episode. Although I can't embed the flash videos from the EduOnDemand site, the episode on similarity is available on YouTube:



THE MECHANICAL UNIVERSE: The same CalTech team which put Project Mathematics! together used the same approach to teach college-level physics in the series, The Mechanical Universe. Below is one of the few episodes which can still be found on YouTube:



ALGEBRA: IN SIMPLEST POSSIBLE TERMS: This series, hosted by Sol Garfunkel, used real-world applications to teach algebra concepts to high schoolers. The same company who put out The Mechanical Universe has also made Algebra: In Simplest Possible Terms available online for free. The general style is the same as the CalTech programs, but with its own distinctly different flavor. I don't have an embeddable YouTube video for this show, but a short clip from episode 11, on circles and parabolas, can be watched online.

Hopefully, you'll explore some of these videos, and learn something new when you do. If you have any favorite creatively-produced educational videos, I'd love to hear about them in the comments below!

Many in the US are enjoying a lazy 4-day weekend as I write this. That being the case, I'll keep the feats relatively simple.

In this post, you'll find out how to easily give others a new perspective by looking at time and space in new ways!

EARTH PHASE FROM THE MOON: If you've practiced working out the moon phase for any date in your head, whether you do the full version, or just memorized how to do it for 1 particular year, this feat is surprisingly simple.

Once you've determined the phase of the moon on a given date, the phase of the Earth as seen from the moon will be exactly the opposite phase! If the moon, as seen from the Earth, is in a full moon, then the Earth, as viewed from the moon, will be a “New Earth” (the Earth will be unlit). If the moon is in a waxing gibbous phase (more than 50% lit, and getting brighter each night), then the Earth, as seen from the moon, will be in a waning crescent phase (the Earth will be less than 50% lit, and getting darker each night).

Why does it work out this way? Take a look at the moon phase diagram below. Pick a phase, and follow that phase's line from the Earth to the moon, and imagine extending it through the moon. Imagine yourself out in space, along that line, looking at the opposite side of the moon that everyone on Earth sees. It's not hard to understand that the moon on this side must be in the opposite phase. If one side is getting brighter, the other side must be getting darker, and vice-versa.

Now, imagine yourself on that same line, but now you're between the Earth and the moon, facing the Earth. The sun is far enough away (90+ million miles!) that it's going to be lighting the opposite side of the moon and the Earth in the same way.



Just as in the original feat, you can verify this with Wolfram Alpha. If someone asks for the moon phase for, say, December 10, 2014, you would use the standard feat to estimate that the moon would be 19 days old (18-20 days old, including the margin of error), so you'd know it's in a waning gibbous phase, which means the moon is more than 50% lit, and getting darker each night.

Conversely, the Earth, as viewed from the moon, must be in a waxing crescent phase, so the Earth is less than 50% lit, and getting brighter each night. Wolfram Alpha can verify this for you.

1 MILLION SECONDS AGO: When you hear large numbers tossed around, it's really hard to get a sense of scale. How big is something like 1 million? To put it into perspective, imagine we're talking about 1 million seconds. When was it 1 million seconds ago?

Determining this isn't hard, especially if you just want to give the correct date. 1 million seconds is roughly 11.5 days. You can work out in your head what day 12 days ago was, or just cheat and use Wolfram Alpha to find out. If your local time is 1:46 PM or before, 1 million seconds ago was 12 days ago. If your local time is 1:47 PM or after, 1 million seconds ago was 11 days ago.

I'm writing this paragraph on December 1st, 2013, at about 11:45 AM local time, so 1 million seconds ago was November 19th, 2013. If I'm asked this afternoon at, say, 3:30 PM when 1 million seconds ago was, I'd say it was November 20, 2013, instead, because that is after 1:47 PM.

If you're interested in giving the exact minute, take the current time, add 13 minutes, then add 10 hours. 1 million seconds before December 1st at 11:45 AM would be November 19th of the same year at 9:58 PM, because 11:45 AM plus 13 minutes is 11:58 AM, and 10 hours after that is 9:58 PM.

If you're challenged to work out the exact second it was 1 million seconds ago, add 13 minutes and 20 seconds before adding the 10 hours. On 1:46:40 PM local time on any given day, 1 million seconds ago was exactly midnight, heading into 11 days ago.

As always, people can verify your answer using Wolfram Alpha.

1 BILLION SECONDS AGO: Since we're talking about large numbers, many people don't realize the difference in scale between 1 million and 1 billion, so when was 1 billion seconds ago?

1 billion seconds is over 31 years ago, so don't try and work out the exact date in your head. For this one, just look it up in Wolfram Alpha. As I write this on December 1, 2013, 1 billion seconds ago was March 25, 1982.

Working out the exact time is even simpler for 1 billion seconds ago, as it happens. First, add 13 minutes (and 20 seconds, if desired), just as before, but subtract 2 hours instead of adding 10 hours. December 1, 2013 at 11:45 AM minus 1 billion seconds is March 25, 1982 at 9:58 AM. Yes, your calculations can be verified with Wolfram Alpha.

You can make older dates like this more vivid by looking up those days on Wolfram Alpha or Wikipedia's year pages. For example, just a quick scan of those pages, I can remember that Danica Patrick was born, the first computer virus was only 2 months old, and the Vietnam Veteran's Memorial in Washington, D.C. would be opened the next day for the very first time.

1 TRILLION SECONDS AGO: 1 trillion seconds ago is the easiest, because that was 31,689 years ago, before modern clocks or calendars existed. This is roughly around 30,000 BCE, so ideas like the bow and arrow were still new, and not a single person was living in Japan yet. Obviously, if you include this, it's more for the sense of scale as compared to 1 million and 1 billion seconds ago.

If you like more mind-blowing changes in perspective, check out my Astronomical Scale post, and be ready for even more surprises!