This post and the next are about handy mnemonics for trigonometry. For both of them, I'm going to assume you're familiar with the unit circle and Pi.

We'll start off with Vi Hart's rant about Pi being wrong. This is a conviction shared by Michael Hartl and Bob Palais, among others:



If the part about Euler's identity confuses you, check out BetterExplained.com's Intuitive Understanding Of Euler’s Formula.

My own solution to this problem is to preserve Pi, and just switch to “diameterians”, a unit of measure equal to 2 radians. Even if we eventually do switch to Tau or diameterians, the switch isn't going to happen anytime soon. Until that time, we need a way to remember facts about the unit circle relating to the way they're taught now.

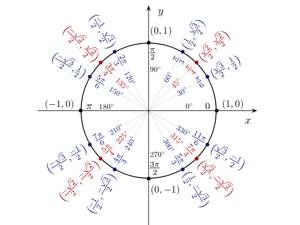
Without knowing it, Vi Hart has inspired an idea in me for a mnemonic to help convert from degrees to radians easily. The trick is to first think about a measure in “pies”, as if you were considering how much Pi is left, and then to think about the measure in Pi.

Let's start with a simple example, that of 180° (degrees). How many radians is that? Well, if you had an actual pie, and only 180° was left, you only have half of a pie. Put more formally, you have:



It may sound ridiculous, but I'm always going to be using the plural phrase, “in pies”, because it's going to help lock in the mnemonic. I'll explain that shortly, but first we need a starting point.

If you're given any multiple of 90°, it's pretty easy to picture these in pies, as it was how many of us were taught:



45° isn't much harder, especially considering that we've already dealt with half of them above. You just have to remember that 45° is 1/8 of a pie. Here are the rest:



The last set of multiples you usually need to know when dealing with the unit circle is 30°, or 1/12 of a circle. Without duplicating any of the above, here are the final fractions:



As you're about to see, if you can convert degrees into a fractions of pies, you're most of the way there.

When changing the word from “pies” to “pi”, we have to chop off half of the letters, don't we? Similarly, we halve to chop the fraction in half. This is the handy mnemonic I promised you earlier. To convert from “in pies” to “in pi” we have to chop off half the letters and half the value of the fraction.

How do we go about this? It's simple, just take the denominator (the bottom number of the fraction), and divide it by two. When dealing with 4ths, 8th or 12ths as above, this will always be possible. Let's try this with 90°:



See? It's easy! Now, in the unit circle, Pi is always put on the top of the fraction, so we should actually do it this way:



Trying this with a tricker number, such as 315°, once you get the fraction in pies, is just as easy:



In short, if you remember to convert from pies to pi by cutting the letters in half, you can remember to cut the number on the bottom of the fraction in half, as well. When dealing with the common amounts of 30°, 45°, 90° and their multiples, this works well.

What happens when you come up with a more unusual angle, such as 216°? Let's try it and find out:



We're still in pies, and we've run into a problem? If we divide by 2 to convert from pies to pi, we'll wind up with 3/2.5! It's one thing to reduce fractions, but that's going a little far.

In this case, we use the alternative approach of doubling the numerator (top number of the fraction). Continuing in this manner, we get:



To help remember which number you double and which number you divide by two, here's another mnemonic: You can make the Lower number Lesser, and the Higher number more Humongous. Alternatively, you could think of making the Lower number Lighter and the Higher number Heavier.

Practice this, and you'll soon have no problem converting degrees to radians in your head. Once you've got this down, you also shouldn't have any problem converting radians to degrees, as well.

Since, in this case, you're going from pi to pies thus doubling the number of letters, you'll be doubling the denominator, as well. Let's try to figure out Pi/12 radians in degrees:



That covers degree and radian conversion in your head. In Sunday's post, you'll learn how to calculate sine and cosine on your fingers! (No, I'm not kidding.)

It's been a long time since the release of both Impuzzibilities and Further Impuzzibilities, but author Jim Steinmeyer has finally released the latest in the series, Subsequent Impuzzibilities. Is it a worthy successor to the previous two books? That's the topic of today's post.

If you're not already familiar with the first books in the Impuzzibilities series, either go buy them now, or at least check out my 2006 review of both books. That way, at least we're starting at the same point.

Subsequent Impuzzibilities should be of interest to Grey Matters readers not only because it's about magic, but especially because the focus is on math-based self-working routines. Because of this focus, you can be amazed by performing the tricks as you read them. You can perform many of them over the phone, as well.

Before I get into the individual tricks, I'd like to mention that the psychology of each presentation is very finely crafted, and should be studied thoroughly as lessons in themselves. This shouldn't be surprising, since Jim Steinmeyer himself is the mind behind some of the greatest illusions and presentations of our time.

The book opens with “Eleven Roads to Heaven”, which is a great example of my point about the presentations. This 11-card routine involves repeating the same sequence of steps several times, which could quickly and easily become boring for the spectator. However, the ingenious presentation here of being able to pick out a face in a crowd the more you see it prevents the spectator's potential boredom.

In the previous book, Further Impuzzibilities, there was a trick called “Automatic Palmistry”, involving absolutely no props other than the spectator's hands. This book's “Fingertip Mindreading” is a good follow-up involving only the fingers of one of the spectator's hands.

One of the big hits of the original Impuzzibilities was the Nine Card Trick, which was popularized by no less than David Copperfield:



In Subsequent Impuzzibilities, continues examining the possibilities of this principle with “The Password Fallacy” and “A Universal Password”. In both of these routines, the routine is played as an example of the performer finding out the spectator's secret (a card name) as if they were hacking a password. I haven't seen this presentation used much in magic, yet it seems such an obvious match for many card location routines. This is where you start getting a sense for the genius of Jim Steinmeyer.

What the “Why didn't I think of that?” password approach is to presentation, the approach used in “Enigmatic Poker” and “Enigmatic Tarot” are to method. These tricks involve a somewhat unusual procedure involving the spectator telling you exactly how to shuffle, while the cards remain under much more control than it would seem. Even though they use exactly the same mixing procedure, their respective presentations justify the strange mixing in two different ways. Already I can see that this is the one thing from the book that will be adapted in myriad ways by magicians in the coming months.

Also written up earlier in the Impuzzibilities series is the “One O'Clock Mystery”, also popularized by David Copperfield:



In a similar vein, the newest book contains a number of similar effects in which several things are seemingly chosen at random, yet the outcome can be predicted in a myriad of amazing ways. “Number, Number, Number” and “A Trip Around the World” are longer routines with surprising punches, while “Force Six” and “Force Ten” are simple enough to print on your business card!

“Deal Three” is a great routine involving a different mixing procedure, this time done by the spectator. It almost seems to be a halfway point between the password routines and “Enigmatic Poker”. The person for whom you're performing can do this seemingly-fair mixing procedure as much as they want, yet you can still control the outcome. I can see this being very deceptive when mixed with the Free Will principle.

The remaining two routines from Subsequent Impuzzibilities I've saved for mentioning at the end because they both play big in their own way. “Ten in Concert” is a 3-phase routine in which the cards themselves repeatedly seem to know about the audience member's choices.

“The Magian Who Fools Himself” plays big because it can be done as a stage routine. All you need is someone from the audience, a deck of cards, a chair for the person to sit on, and something to hold the cards, such as a glass. In this effect, the spectator plays the part of the magician, and causes a card to vanish from one place and reappear in another. It's a re-working of the principle in “Teleportation”, and is an excellent lesson in why not to stop thinking about a routine.

Because these tricks are self-working, many may dismiss them. Not only will those who dismiss these tricks lose out on the presentations already there, but they won't see the potential of adapting these pieces to themselves. Also, there's nothing wrong with adding the occasional sleight or subtlety to improve them. David Copperfield's videos above are a good example of adapting and adding to routines like these.

In short, I recommend Subsequent Impuzzibilities highly, as well as all the Impuzzibilities books, not only for the tricks in the book, but for what you can learn about presentation, how to think about magic, and keeping things simple.

–––––––––––––––––––––––––––––––––––––––––––––––––––

Answer to Pi Day Magic 2 puzzle:
4C, 4D, KH, 9H - This arrangement could be used to cue the 6C.

Back in the Grey Matters' 6th Blogiversary post, I mentioned that James Grime had something up his sleeve for this year's Pi Day, as well. He slightly missed the deadline, but has delivered nonetheless.

Let's start with James Grime's own rather unusual description of this year's Pi Day trick. He starts the video with an apology for the delay, and then goes on to explain the trick with puppets (yes, puppets):



To answer your first two questions, yes this is a real trick, and yes, the app is real. The app is called Pi Day Magic (iTunes Link), and is available for free on the iPhone, iPod Touch, and the iPad (running in 2× mode)! At this writing, the app is expected to be released for other smartphones soon.

Knowledgeable magicians and longtime Grey Matters readers will recognize this trick as the Fitch Cheney Card Trick. In that 2008 write-up, I tried to write it step by step, so you can understand the trick completely in as clear a manner as possible. The secret is also explained more briefly in the app itself.

Normally, performing this trick requires two people. Using the app instead, the smartphone or tablet you're using takes the role of the secret helper. I do believe using a secret helper is more effective here, because it more effectively takes the mathematical air off of the trick.

That said, the app is a great way to practice the trick. As the performer, you can practice reading the order of the cards (which you still need a second person to select) to get the knowledge of the 5th card. As the secret helper, you can also use it to help make sure that the cards are being arranged in the proper manner.

Practicing as the secret helper with the app, however, can be a little tricky. It's often possible to arrange the cards in more than one way, and the app will only arrange the cards in one of the possible ways. This doesn't necessarily mean that you arranged the cards wrong, just that the program chose a different target card.

If you've made the effort to learn this trick as described in the app or my post, here's a puzzle for you (no fair using the app to figure this out): Take out the same cards used in the video above (KH, 6C, 4C, 4D, and 9H). Arrange the cards so that another card, instead of the King of Hearts, is face down. Which card is face down, and how are the other cards arranged to communicate it?

Post your answer in the comments, and I'll acknowledge the first right answer I receive. If no one gets it, I'll post the answer at the end of Sunday's blog post (March 27th).

As much as I teach real memory feats, I'm not above the occasional fake demonstration. Many of them, like the one taught on Scam School in the video below, are quite ingenious in their methods.

If you don't believe me, just check out the 15 pages under Simulated Memory Demonstrations in my Memory Effects list.

I've run across several fake memory demonstrations that were less than convincing, so I have an appreciation for the simple and direct methods. Watch the presentation up to about the 2:50 mark, and see if you can figure out the method.



If you're not familiar with the key card concept Brian mentions, here's the Scam School episode in which he teaches it.

Any thoughts yet? OK, go ahead and watch the rest of the video for the method now.

This type of presentation was originated by Morris “Moe” Seidenstein (1909-2003), who first published it in his book Moe's Miracles as “Move A Card”. The original method relied more on a trained memory.

As an alternative to real memory work, I think the use of two key cards to highlight a range of cards in ingenious. The video is a little unclear on the exact process of returning the card to the deck between the key cards, but a quick reading of the Scam School forum thread for this episode will help clear up that confusion.

The last comment on that thread does bring up the possibility of having 8 to 10 cards between your key cards. How do you handle that? This is where a legitimate trained memory comes in handy, but the work is simpler than you may think. If you practice the Link System in conjunction with Bob Farmer's playing card mnemonics, facing 8 to 10 cards or more will be a snap. If you want to practice this way, try shuffling more than once. You might be surprised just how many cards you can remember!

As a side note, this does bring up how truly randomizing a standard riffle shuffle can be. Both the recent Gilbreath Principle episode and episode 31, titled How to Predict the Future!, teach you how to make surprisingly specific statements about a legitimately shuffled deck, even without looking at it after the shuffle.

Play around with this, and tell me about your experiences and ideas in the comments.

So, how do I kick off my 7th year of blogging at Grey Matters? By creating a mobile version of this site!

If you're visiting Grey Matters on a mobile phone, you've already noticed the difference. If the site detects your mobile device, it will automatically redirect to the mobile blog for an easier reading experience.

Yes, there has been a Mobile button at the top of this page since the new design was added. Originally, it went to a separate mobile blog that never really developed (and has now been deleted).

Now, however, that Mobile button has a brand new function! On both this blog and the video section, clicking the Mobile button will immediately take you to the brand new mobile blog. Further, if you're looking at an individual post,as you are now, a monthly archive page, such as this one, or a label page like this one, clicking the Mobile button will take you to their corresponding mobile pages.

If you decide you prefer the standard web version, simply scroll to the bottom of any mobile page, and click on View web version.

Due to the use of special interactive features such as tabs and quizzes, the Mental Gym and Presentation don't have mobile versions. Clicking on the Mobile button anywhere in these sections will simply take you to the mobile version of the blog.

Just as in the regular blog, you can view and post comments below each individual mobile post, as well as go back and forth to newer and older posts.

Don't forget that the web version is also also designed to be accessible by smartphones and tablets.

If you have any comments, criticisms, or suggestions regarding the new mobile blog, I'd love to hear about them in the comments!

Can you believe it? Today, Grey Matters is 6 years old! It's expanded, grown, and changed beyond even what I expected when I started it!

It's also Pi Day (3/14) and Albert Einstein's birthday, so let's celebrate in a geeky manner!

The best way to start? Check out all the Pi entries here on the blog, in the Mental Gym, and in the Video section. If you haven't guessed already, it's no coincidence that I started Grey Matters on Pi Day.

Even when going beyond this site, it's amazing where you can find Pi. As proof, take a look at the Mathematical Association of America's collection of Pi photos!

Even on Pi Day, we should consider the fact that Pi may be wrong. Here's Vi Hart to explain the advantages of Tau over Pi:



Futility Closet introduced me to Emma Rounds' “Plane Geometry”, a mathematical parody of Lewis Carroll's Jabberwocky in which Pi earns an honored place:

‘Twas Euclid, and the theorem pi
Did plane and solid in the text,
All parallel were the radii,
And the ang-gulls convex’d.

“Beware the Wentworth-Smith, my son,
And the Loci that vacillate;
Beware the Axiom, and shun
The faithless Postulate.”

He took his Waterman in hand;
Long time the proper proof he sought;
Then rested he by the XYZ
And sat awhile in thought.

And as in inverse thought he sat
A brilliant proof, in lines of flame,
All neat and trim, it came to him,
Tangenting as it came.

“AB, CD,” reflected he–
The Waterman went snicker-snack–
He Q.E.D.-ed, and, proud indeed,
He trapezoided back.

“And hast thou proved the 29th?
Come to my arms, my radius boy!
O good for you! O one point two!”
He rhombused in his joy.

‘Twas Euclid, and the theorem pi
Did plane and solid in the text;
All parallel were the radii,
And the ang-gulls convex’d.

It seems the promise of spring is making everyone more productive. Let's see what's going on in this edition of snippets.

Just a quick note before we begin – Instead of my usual Sunday post, my next post will be on Monday. Why? It's March 14th or 3/14. That means it's Pi Day, Albert Einstein's birthday, and Grey Matters' 6th blogiversary!

• If you enjoy MAGIC Magazine, but wish their database index didn't end at October 2009, I've been working hard to make sure you got your wish! Over at the Magic Cafe, I've been adding unofficial database index additions. At this writing, updates are available for all the issues up to and including March 2011. They're posted in CSV format, which can be loaded in by most database or spreadsheet programs.

• Speaking of magic magazines, both MAGIC and Genii just made several major computer-related announcements!

MAGIC Magazine now has an app that lets you purchase and read the magazine on the iPad! At this writing, the January, February, and March 2011 issues are already available on the app. It also includes a free video by Mac King showing you how to use the app.

Genii is working on it's own digital version, which has been dubbed iGenii. As a preview, there's excerpts of four iGenii issues available online for free! Even more impressive is their recent announcement concerning their digital archive. Starting this fall, any and all subscribers to Genii receive free access to their digital archive! Considering this includes issues going back to 1936, this is a VERY good deal for the price.

• For those of you who take advantage of my Memorized Deck Online Toolbox post, I've gone through and updated the links and removed dead links. I also added a brand new addition, Mark Storms' online 52-item Peg List quiz. These are useful for providing imagery for the position number, and setting it up as an online quiz makes it even more convenient to learn!

• A couple of Grey Matters favorites have both been hard at work since we last visited them, so let's go check in.

Scam School has had several great episodes lately. Regular Grey Matters readers will espeically enjoy the Mind-Blowing iPhone Calculator Trick and the brand-new playing card memory feat episodes. However, don't be afraid to explore the rest of them.

The other Grey Matters favorite who has been hard at work is Werner Miller. If you're unfamiliar with his work, check out his generous contributions to Grey Matters for free. Over at Lybrary.com, he now has four ebooks comprising his contributions to magazines over the years: Da Capo 1, Da Capo 2, Da Capo 3, Da Capo 4. These are the latest in his line of English and German ebooks.

That's all for now. See you on Pi Day!

Granted, my list of sites to learn the basics of memory technique is handy, but the pages are mostly text and illustrations.

I decided it was time to add video instruction for memory techniques!

I've spent the past few days digging through YouTube videos relating to the various memory techniques, and grouping them into playlists. I tried to order them from simpler descriptions to more complete lectures. I've already added these to the memory basics post for regular access.

The results are below, with the titles linking to their respective YouTube playlist pages:


Memory Technique 1: Beginning Systems

I originally posted the Day of the Week For Any Date tutorial back in 1998. Over the years, I've learned a few tips and tricks to improve both the method itself and the teaching.

As a result, I decided to develop a completely new and revised Day of the Week For Any Date tutorial! What's new about it?

The first major change I made was to eliminate the requirement of having to study and practice other memory systems first. Mnemonics are still used, but they're simpler, shorter, and don't require previous knowledge of any memory techniques.

The lessons are also done gradually, so that you can learn at your own pace. After introducing the approach and teaching weekday and month codes, you immediately start working with the years 2000-2003 to get the basic idea of the formula down.

That immediate application of the formula necessitated two changes of it's own. First, I've started using the 21st century (2000-2099) as the basic century. The original tutorial used the 20th century (1900-1999) as the basic century.

Second, instead of teaching about leap years at the end of the tutorial, they're now an integral part of learning how to do this feat. When learning about the formula, you quickly learn how to handle leap years for dealing with January and February dates in 2000. The very next step is learning mnemonics for the first 7 leap years, and then how to handle the remaining leap years without any more mnemonics!

Once you have all the leap years down, the remaining years are easily learned, too. Handling other centuries can be picked up quickly, as well.

Of course, none of these steps are effective unless you practice them. All this re-working of the method naturally required a completely new quiz. Seeing the quiz buttons, you quickly get a feel for the pace of the lessons: Learn and practice codes, then learn and practice dates with them, move on to the next set of codes, and repeat.

It may seem slower, but the more gradual nature of the new tutorial should make it easier to learn and less intimidating.

Have you tried to learn this feat before? Do you like this version of the tutorial? I'd love to hear your comments and criticisms in the comments!