This week's post is a day early because today is Tau Day!

Tau, for those not familiar with it, is the mathematical constant equal to 2 × π (Pi), or roughly 6.28, and is represent by the greek letter Tau: τ. Today (6/28), we're going to look at the internet battle that's erupted over π versus τ since 2010.

The opening salvos in this year's battle were already launched back on Pi Day by DNews, with their Is Tau Better Than Pi? video:



3 days ago, Scientific American continued with their post, “Why Tau Trumps Pi”. Just 1 day later, prooffreader.com jumped in with Pi vs. tau: Ultimate Smackdown.

Starting at about 2:40 into the DNews video above, they come very close to a good answer. Yes, geometry and trigonometry rely heavily on 2π, and in those cases τ makes more sense, especially when it comes to concepts like τ radians in a circle. However, π has plenty of uses beyond those subjects on its own.

I'm in favor of adopting τ as a commonly-used constant, but not as a replacement for π. Talking about τ versus π to me is like getting in a heated argument over degrees versus radians. Which one is better? The answer can be degrees OR radians, depending on the context of the problem at hand. Should we use base 10, base 2, or base e in logarithms and exponents? Again, the answer depends on the context.

Kalid Azad of BetterExplained.com has 3 questions that can make even the toughest math concepts understandable: What relationship does this model represent? What real-world items share this relationship? Does that relationship make sense to me? In fact, as James Sedgwick points out in his essay, “The Meaning of Life”, you only have meaning if you have a relationship set in a context.

Yes, the endless internet battles over Pi versus Tau can be fun, but when it comes down to the important aspects, I believe we should focus on solving the problem at hand, and using the most effective tools. Besides, if we only keep one or the other, that's one less geeky holiday to celebrate in the year.

Happy Tau Day!

Back in the 1960s, Popular Science frequently included inserts focusing on topics of interest to their readers.

Since this was a science magazine in the days before pocket calculators were common, there were many times that the inserts focused on making mental calculations easier. Thanks to Google books, these are available once again!

Our first jump takes us all the way back to the January 1961 issue, which features excerpts from Lester Meyer's book, High Speed Math. The great body of the 14-page book digest focuses on shortcuts for multiplication, but towards the end, there are tips about addition, subtraction, division, and knowing when to use given shortcuts:



Later that same year, in November, they returned to this idea with an insert called “Math and Memory Short Cuts”. This particular book, for some unknown reason, was scanned in reverse page order. Despite that minor flaw, the insert is still readable. Instead of straight math shortcuts, this insert focused on math and memory techniques that were handy in the home workshop.



Popular Science returned to pure math shortcuts in December 1964, with their book digest of Isaac Asimov's Quick and Easy Math. This 7-page feature was arranged in the more traditional order of tips for addition, subtraction, multiplication, and division. If you're only familiar with Asimov's fiction writing, you might be surprised at the clarity he can bring to instructional writing:



Our final insert comes from the March 1967 issue, in an insert titled “High-Speed Math Short Cuts” This one is dedicated almost entirely to quick multiplication and division techniques, including a handy reference table giving the results of 1000 ÷ n for every integer n from 1 to 999, plus tips on using the table to handle larger numbers!



Obviously, these techniques were far more essential in the days before pocket calculators were common. However, the techniques are still useful, so they're fun to explore. You never know when and where you'll find that perfect shortcut for frequently-encountered problems!

June's snippets are ready!

This month, we're going back to some favorite topics, and provide some updates and new approaches.

• Let's start the snippets with our old friend Nim. The Puzzles.com site features a few Nim-based challenges. The Classic Nim challenge shouldn't pose any difficulty for regular Grey Matters readers.

Square Nim is a bit different. At first glance, it might seem to be identical to Chocolate Nim, but there are important differences to which you need to pay attention.

Circle Nim is a bit of a double challenge. First, you may need to try and figure it out. Second, the solution is images-only. Once you realize that different pairs of images are referring to games involving odd or even number starting points, it shouldn't be too hard to understand.

• Check out the Vanishing Leprechaun trick in the following video:



These are what are known as geometric vanishes, and can be explored further in places such as Archimedes' Laboratory and the Games column in the June 1989 issue of OMNI Magazine.

Mathematician Donald Knuth put his own spin on these by using the format to compose a poem called Disappearances. If you'd like to see just how challenging it is to compose a poem in geometric vanish form, you can try making your own in Mariano Tomatis' Magic Poems Editor.

• Back in July 2011, I wrote a post about hyperthymesia, a condition in which details about every day of one's life are remembered vividly. That post included a 60 Minutes report about several people with hyperthymesia, including Taxi star Marilu Henner. Earlier this year, 60 Minutes returned to the topic with a new story dubbed Memory Wizards. This updated report is definitely worth a look!

• If you're comfortable squaring 2-digit numbers, as taught in the Mental Gym, and you think you're ready to move on to squaring 3-digit numbers, try this startlingly simple technique from Mind Math:



That's all for June's snippets. I hope you have fun exploring them!

Today, I'm going to take you on a journey. It's a sort of imaginary journey, but not in the way that you may think.

This is a mathematical journey, and if you can grasp where we start, I think you'll enjoy where we wind up.

There are two important concepts with which you'll need to be familiar before we begin. The first is the idea of imaginary and complex numbers, which are explained intuitively in A Visual, Intuitive Guide to Imaginary Numbers. The other concept is the number e, as explained in An Intuitive Guide To Exponential Functions & e. Take your time, if needed, to understand these concepts, and the rest of this post will be well worth the time spent.

We'll start simply, with a right triangle that's half as high as it is long (via Desmos.com). The coordinates of the vertices in Cartesian coordinates are (0,0), (1,0), and (1, 0.5). Converting these to complex numbers, they respectively become as 0 (0 + 0i), 1 (1 + 0i), and 1 + 0.5i. As you can see, converting between complex numbers and Cartesian coordinates requires little effort.

Just for fun, let's see what happens if we take this 1 + 0.5i right triangle, and take it the integer powers from 1 to 10. Wolfram|Alpha calculates the answers to (1 + 0.5i)ⁿ quickly, for values of n from 1 to 10, but the answers are just complex numbers, with no real apparent meaning.

Desmos.com can't handle complex numbers, but can handle tables of Cartesian coordinates, so if we use those answers, and draw the corresponding triangles, we get a very visual interpretation of these complex numbers!



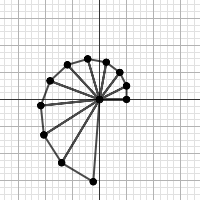
What we get is a series of larger and larger right triangles! Think about why this is, using our old friend, the Pythagorean theorem. The horizontal leg is 1 unit, the vertical leg is 0.5 units, so the Pythagorean theorem tells us that the hypotenuse of that first triangle is about 1.11803 units long. That's also the base of the next right triangle.

The distance formula can be used to work out the height of the new triangle. It starts at (1, 0.5) and ends at (0.75, 1), so we plug the numbers in to get d = √(0.75 - 1)² + (1 - 0.5)² = √(-0.25)² + (0.5)² = √0.0625 + 0.25 = √0.3125 ≈ 0.559017.

Notice that, if you double 0.559017, you get 1.11803. That's not a coincidence. What you're getting is a series of triangles with progressively longer legs, and whose heights will always be half of that length. In short, (1 + 0.5i)ⁿ builds n right triangles with a 1:0.5 (or 2:1) ratio for the legs, and hypotenuses who lengths grow in accordance with the Pythagorean theorem!

Now, the formula (1 + 0.5i)ⁿ looks a little like the part of the formula for e. What would happen if we changed it to (1 + ^0.5i⁄_n)ⁿ? Let's look at this step by step.

With just 1 power, and therefore just 1 triangle, the formula gives us 1 + 0.5i, not surprisingly. This gives us our original right triangle.

Next, let's change the power to 2, and step through the formula twice to get 2 triangles. Wolfram|Alpha returns 1+0.25i and 0.9375+0.5i. We still get 2 triangles, but now they occupy about the same height as the previous single triangle!



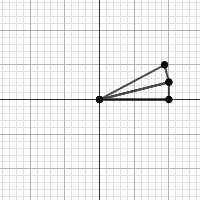
Sure enough, if we take 3 steps through the 3rd power, we get 3 right triangles with about the same total height as the others.



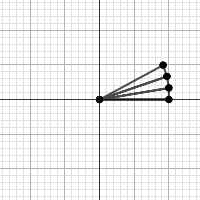
This should make sense, especially as this approach was inspired by e. Remember how the change in e gets smaller and smaller as bigger numbers are used for n? Just like e packs smaller and smaller numbers in the same numeric space, our right triangles are packing more and more triangles in the same space on the graph!

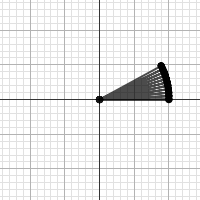
Yes, if we take this approach to the 10th power and take 10 steps through it, we get 10 right triangles packed into the same space.



Let's take a closer comparison of this formula to e. First, the actual definition of e is:



We didn't take that limit into consideration in our triangle demonstration. What happens as our number of triangles get closer and closer to infinity? There will be more and more, so the height will get closer and closer to 0 units. If the height gets closer to 0, the Pythagorean theorem tells use that the hypotenuse will get closer and closer to the length of the original leg, which is 1 unit.

If you can picture infinitely many triangles packed into that same space, you can see that it would almost be like the lengths of the long legs would all be 1 unit, so it would effectively be a perfect arc!

The more general definition of e defines what happens when e is taken to a power:



So, if we apply that limit to our original complex equation, (1 + ^0.5i⁄_n)ⁿ, that means the infinitely many right triangles yields the same result as e^0.5i! Remember that taking our formula to the 10th power ended in 0.888809+0.485079i, so you shouldn't be surprised to see that e^0.5i roughly equals 0.877583 + 0.479426i.

Wolfram|Alpha's Arg[] command takes a complex number and returns the equivalent in radians. Entering Arg[0.877583 + 0.479426i] gives us the rather interesting result of 0.5 radians!

Let's think about this. Plotting out an infinite number of triangles of the form (1 + ^0.5i⁄_n)ⁿ, the equivalent of e^0.5i, results in an arc that's 0.5 radians long.

Yep, we're actually getting visual and mathematical proof that e^ix will result in an arc that's x radians long! If you understand trigonometry, this means you can use sine and cosine to work out the same point calculated by e^ix, which is exactly what Euler's formula says!

Yep, since Pi radians is half a circle, then our formula becomes e^iπ, which is Euler's famous identity! Since the cosine of π = -1 and the sine of π = 0, it works out -1 + 0i, or simply -1.

Did you ever think that playing around with a few triangles would ever lead you to an understanding of e^iπ = -1?